The fractal I am choosing to print is called the Hilbert Curve. According to the post on MathWorld, it was first described by David Hilbert in 1891. This curve is a variation of the Peano-curves created by Giuseppe Peano in 1890.
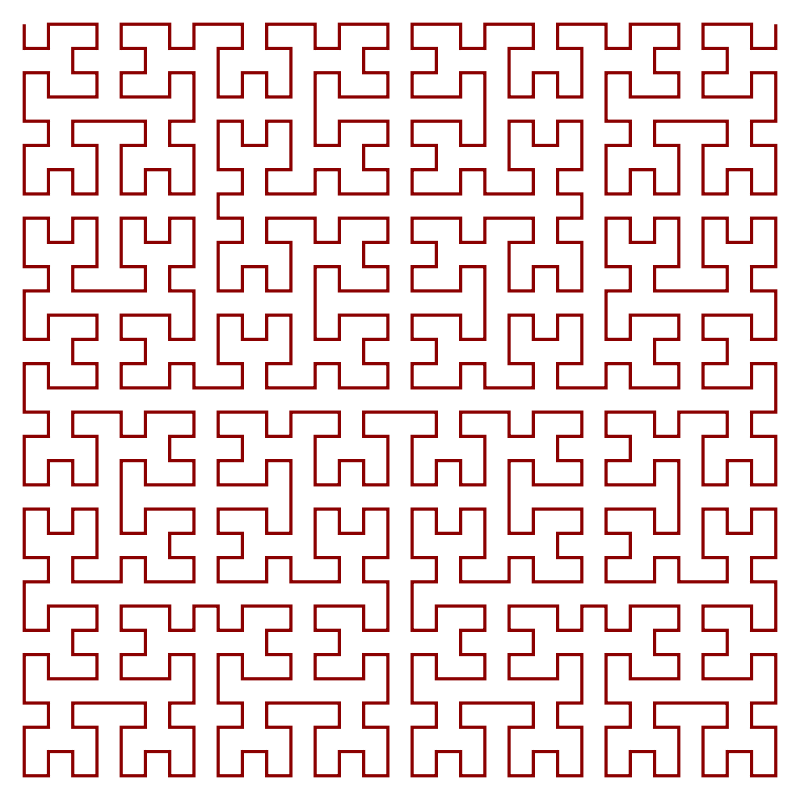
This is a space-filling fractal, like the dragon curve. The more iterations you do, the bigger it’ll get. Eventually, it’ll begin to fill space. I don’t exactly know how or why that happens, but it looks pretty cool. I know that a fractal is just a pattern that repeats infinitely, but I don’t know how someone can create one. In the Wikipedia article here, you can see the progression of the fractal from its first order to its 3-dimensional form.
I’m choosing to print this because it seems relatively easy, and it reminded me of the dragon curve. The model I plan on recreating was done by I_am_me on Thingiverse. However, mine will be flat instead of curved because I don’t know how to make things curved on the printers yet, and that seems like it would be more complicated than I can handle. If the curved print turns out to be too complicated, I will use the model done by pmoews on Thingiverse instead. I think the Hilbert Curve is printable because it seems similar to the Dragon Curve, and we all printed those in class. In addition, it doesn’t have in depth and intricate designs on it.
Printing process
My group and I printed my fractal and a hexagon fractal on the first day. The print wasn’t really that difficult. It was definitely more simple than I thought it was going to be. One thing that surprised me about my fractal was that it is not a closed shape; it’s a continuous line! Here’s a picture:
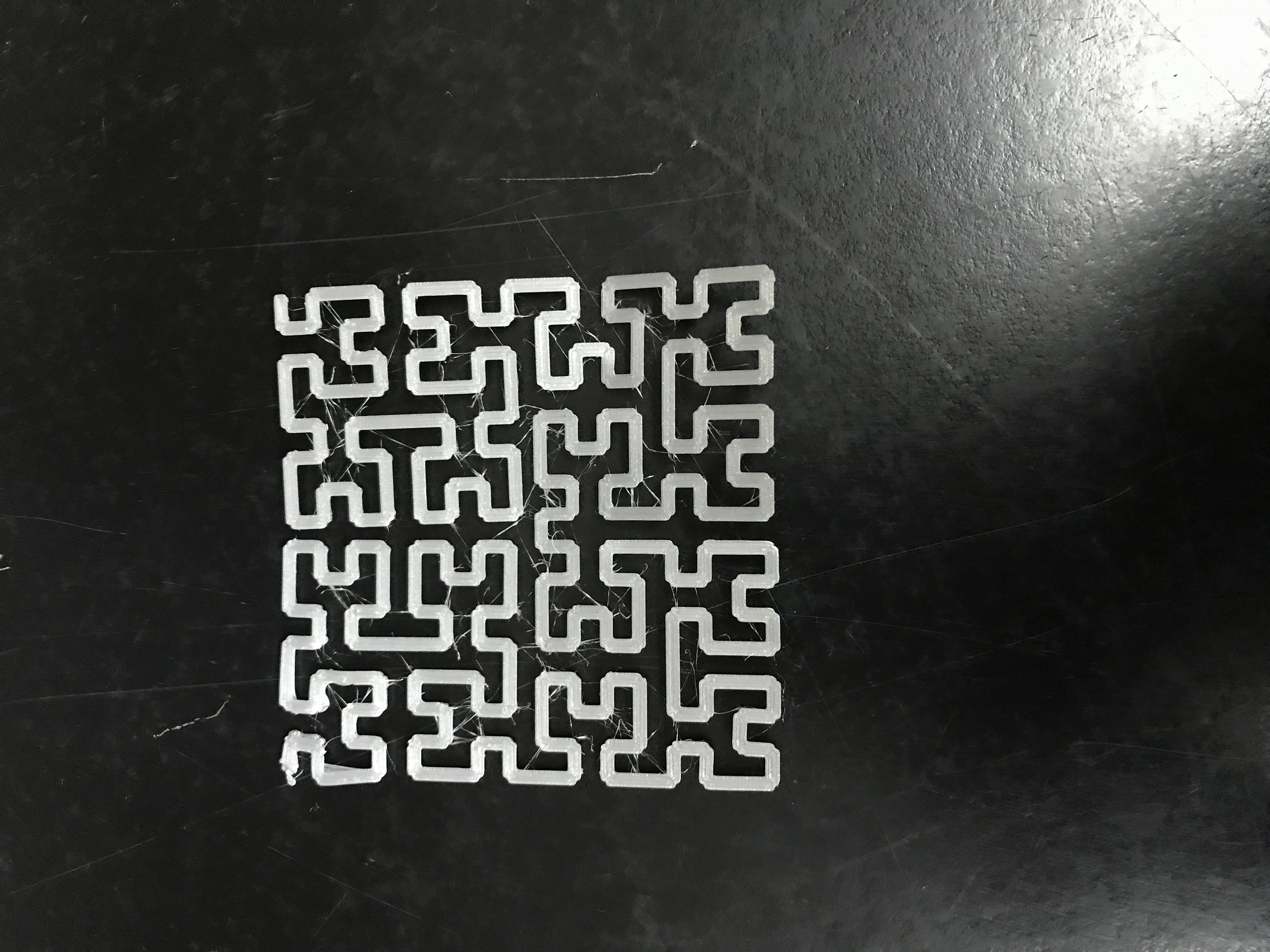
It took a little bit longer than the class period lasted, so we picked it up the next day and ended up printing another one. I also uploaded this picture to thingiverse under the “I made it” link!