Sierpinski Triangle

For my first fractal I would like to print a Sierpinski Triangle because of how neat the concept is. It’s so cool that just repeating a simple shape can create such a complex, beautiful design.

According to Wikipedia, Sierpinski Triangle was discovered by Waclaw Sierpinski in 1915. It is classified as not only a fractal but also as an attractive fixed set, which is a mathematical concept I do not yet understand. A Sierpinski Triangle is created by starting with an equilateral triangle and then subdividing it into smaller equilateral triangles. Simply, you first start with cutting an upside down triangle out of the center of a triangle, then proceed to do the same with the other smaller equilateral triangles that are created from it. There is infinite repetitions of this process and with every repetition/step the design becomes more complex. It is able to be reproduced at any magnification. Here is a picture also from Wikipedia that shows the fractal’s advancement up to level 5.

This Youtube video, Fractal Zoom Sierpinski Gasket by 73x137test demonstrates the infinite property of the Sierpinski’s Triangle (Sierpinski’s Gasket is a synonymous term for it) and how complex the fractal becomes with increased repetition of the triangle pattern.

Though I am not very experienced with 3D printing and am unsure of the exact scale of the printer, I found this model on Thingiverse, Recursive Triangle made by hdelgad2 in OpenScad. Though the creator does not list the dimensions it seems like it would be feasible to print because it does not look too big viewing it in Thingiview. It also might be able to be altered, which I think 50mm across is a reasonable size for the printers and for the shape. However, I am still unsure about the size of the printer and am getting used to exploring Thingiverse. Therefore I am not confident that this is reasonable because I do not know how long it would take to print something like this. If the design is too complicated to print I also discovered that the same creator also built the Reclusive Triangle with less levels to it. So if the first one is too detailed I could print out the one that is more simple which is another reason why I liked hdelgad2’s model specifically.

I think that the design the Sierpinski Triangle is really unique. It is so interesting that no matter how far you zoom in it makes the same pattern and you can continue to replicate it infinitely. I think this would be a really neat fractal to print.

 

3D Printing Results: Printing the Recursive Triangle by hdelgad2 from Thingiverse was much easier than I anticipated. I thought because of the details in the model with more levels I wouldn’t have enough time to print it but it was able to print within 45 minutes! What I also learned by listening to my classmate’s presentations was that the repeating pattern that creates Sierpinski’s Triangle can be used with different shapes to create other fractals, like Sierpinski’s carpet, which I think is really neat. I also learned from a classmate who also printed Sierpinski’s Triangle that after the completion of the first triangle the other triangles are drawn in threes tangent to it, and the repetition of this is what gives it it’s space filling property.

Here is the print of my fractal which printed really nicely:

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

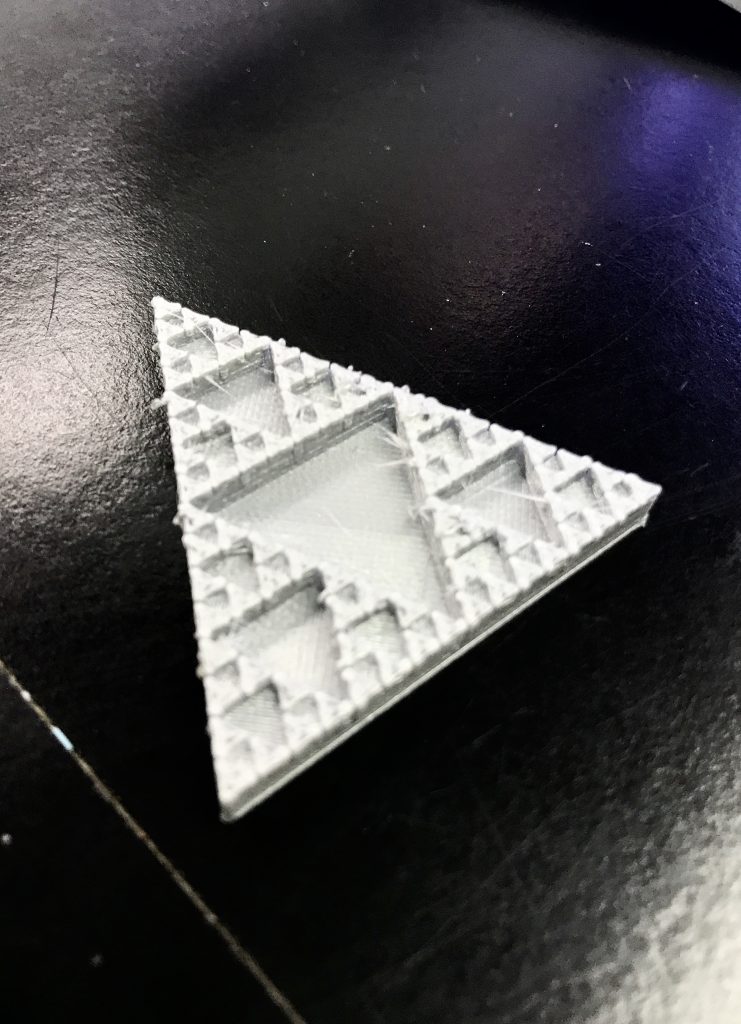
Make:

If you would also like to try and print out this neat model of a Sierpinski Triangle, check out hdelgad2’s model by clicking on this link!

https://www.thingiverse.com/thing:1465806