Sierpinski Carpet

For my first fractal, I would love to try printing a Sierpinski Carpet. Not only am I beginner on the front of 3D printing, but I am also very fascinated by minimalist design. Illustrative Mathematics states that this is a fractal whose area is zero, yet whose perimeter is infinite! Finding the perimeter can be solvable through exponential notation, though I do need to research more and understand why/how that’s possible. In simpler terms, according to Wikipedia, the Sierpinski Carpet is created by cutting a shape into 9 sub congruent equal squares, and then removing the middle square. This is a similar design technique to the Sierpinski Triangle, which involves a similar process but for triangles, or the Menger Sponge, a 3D cube version of the Sierpinski carpet.

I would like to try using this Sierpinski Carpet design by Chevron42 for my first print. Although the print may be a bit complicated and bigger (and I’d like to explore how I can minimize it), I think I can get it down to 50mm. Plus, it lends itself perfectly to dyeing, which would be perfect to use as a print for other things (wall decals, etc.)

In addition, I found this video of an online designer making a Sierpinski Carpet, which shows a pretty solid way to see the simplicity of going deeper in the shape.

3D Printing Results

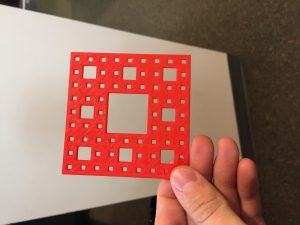
The printing results were nothing short of positive. The fractal printed on the Ultimaker without error or fault. It took about 50 minutes in total (to create, mold, heat, cool down). Compared to other fractals in my group, I believe mine printed the smoothest and the most efficiently (the design is pretty simple after all). I am very proud of the fractal I printed. In addition, I was reading more into the Sierpinski Carpet, and I found out that the fractal has a special relationship with Brownian motion (the seemingly-random motion of gas and liquid particles hitting each other in a space). According to Wikipedia, the Brownian motion on Sierpinski carpets actually diffuses at a slower rate than an unrestricted random walk (Brownian motion). In addition, while random walks reach a mean distance proportional to √n after n steps, the Brownian motion on a discrete Sierpinski carpet reaches only a mean distance proportional to ^β√n for some β > 2, with β representing “the change in the criterion Y per one-unit change in the value of the associated predictor X.” I am not one hundred percent sure of the meaning, but I do believe it is related to regression analysis.